M-ary Signal-Set

The following three signals are used to construct a signal set for transmission eight equally likely signals over an additive white Gaussian noise channel with spectral height 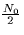$\frac{N_0}{2}$:

Specifically, the eight signals are constructed as linear combinations of the three signals above:

$$s_{lmn}(t) = l \phi_1(t) + m \phi_2(t) + n \phi_3(t),$$

where each of l, m, and n can be either equal to zero or one.

1.

Sketch and accurately label all eight possible signals.

2.

Draw and accurately label a block-diagram of the receiver which minimizes the probability of error for this signal set.

3.

Draw and accurately label the the signal set in an appropriately chosen signal space. Indicate the decision boundaries and state clearly which basis functions span your signal space.

4.

Find the probability of error the optimum receiver achieves with this signal set.

5.

Give a different signal set which achieves the same probability of error as the signal set above.