Binary Transmission in the Presence of a Jammer

In hostile environments an important problem is to overcome the effects of a hostile transmitter that tries to interfere with the communication between the friendly transmitter and receiver. Such a hostile transmitter is often called a jammer. In this problem we investigate how carefully designed signals can help overcome the impact of certain types of jammers.

Specifically, the following two signal sets are under consideration for transmitting equally likely messages over an additive white Gaussian noise channel with spectral height 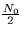$\frac{N_0}{2}$:

  

Figure 1: Signal set 1

  

Figure 2: Signal set 2

1.

Assuming that no jammer is present, draw block diagrams of the receivers which minimize the probability of error for each of the two signal sets above.

2.

For each of the two signal sets above find the minimum probability of error.

3.

Assume now that a jammer transmits a constant signal with amplitude 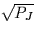$\sqrt{P_J}$, hence the received signal is the sum of the friendly transmitter's signal, additive white Gaussian noise, and the jammer's signal. If your receiver from part (a) is used, what is the probability of error achieved with signal set 1?

4.

What is the probability of error achieved with signal set 2 by your receiver from part (a) in the presence of the jammer?

5.

Is one signal set more robust to the jammer's interference than the other. Why or why not?