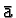Next: Example: A one-error correcting
Up: Electrical Error-Correction CodingSeptember 28,
Previous: Electrical Error-Correction CodingSeptember 28,
- Before we can look at more powerful error correction codes, we need to
look at some basic ways of computing with binaries.
- The following four operations with binary numbers are elementary.
- XOR (exclusive or)
| a |
b |
a 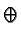 b
b |
| 0 |
0 |
0 |
| 0 |
1 |
1 |
| 1 |
0 |
1 |
| 1 |
1 |
0 |
- OR
| a |
b |
a + b |
| 0 |
0 |
0 |
| 0 |
1 |
1 |
| 1 |
0 |
1 |
| 1 |
1 |
1 |
- AND
| a |
b |
a 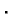 b
b |
| 0 |
0 |
0 |
| 0 |
1 |
0 |
| 1 |
0 |
0 |
| 1 |
1 |
1 |
- NOT (inverse)
| a |
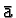 |
| 0 |
1 |
| 1 |
0 |
- The XOR operation is particularly important in connection with error
correction coding.



Next: Example: A one-error correcting
Up: Electrical Error-Correction CodingSeptember 28,
Previous: Electrical Error-Correction CodingSeptember 28,
Prof. Bernd-Peter Paris
1998-12-14
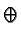 b
b b
b