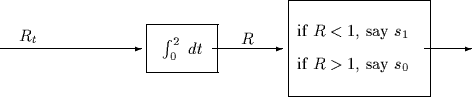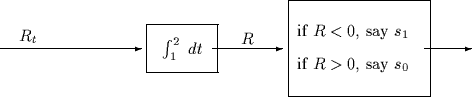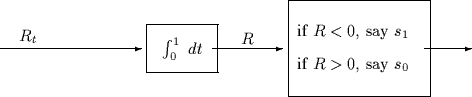Next: Binary Signal Sets Up: Collected Problems Previous: Problem 24

Problem 25

The following signals are used to transmit equally likely messages over a channel corrupted by additive, white Gaussian noise of spectral height 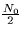$\frac{N_0}{2}$ :

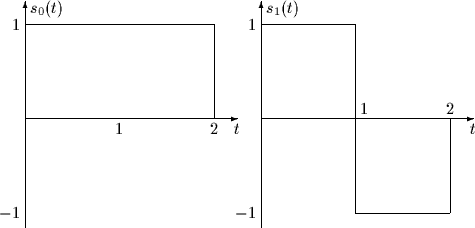
$\begin{picture} (100,50) \setlength {\unitlength}{1mm} \multiput(5,25)(50,0){... ...ine(1,0){20}} \put(75,5){\line(0,1){40}} \put(95,5){\line(0,1){20}}\end{picture}$

Compute the probability of error attained by the following receivers.

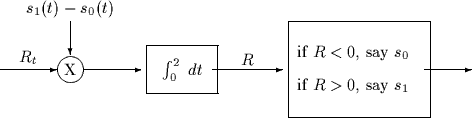
1.

$\begin{picture} (100,30) \setlength {\unitlength}{1mm} \put(0,10){\vector(1,0... ...makebox(30,7){if $R < 0$, say $s_0$}} \put(90,10){\vector(1,0){10}}\end{picture}$

2.

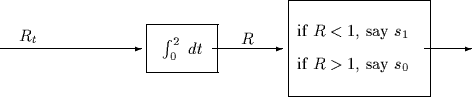
$\begin{picture} (100,30) \setlength {\unitlength}{1mm} \put(0,10){\vector(1,0... ...makebox(30,7){if $R < 1$, say $s_1$}} \put(90,10){\vector(1,0){10}}\end{picture}$

3.

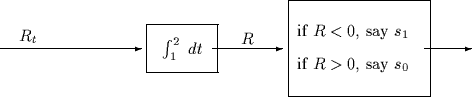
$\begin{picture} (100,30) \setlength {\unitlength}{1mm} \put(0,10){\vector(1,0... ...makebox(30,7){if $R < 0$, say $s_1$}} \put(90,10){\vector(1,0){10}}\end{picture}$

4.

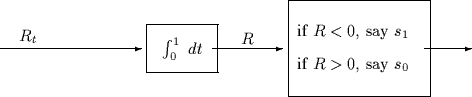
$\begin{picture} (100,30) \setlength {\unitlength}{1mm} \put(0,10){\vector(1,0... ...makebox(30,7){if $R < 0$, say $s_1$}} \put(90,10){\vector(1,0){10}}\end{picture}$

Prof. Bernd-Peter Paris
3/3/1998